Environmental conditions fluctuate on all time scales. Often, those fluctuations take the form of disturbances: relatively discrete events, ranging from footfalls to hurricanes, that harm the affected organisms. In other cases, species may differ in both absolute and relative terms in how they respond to environmental fluctuations: what’s bad for one species may be good (or less bad) for another. Disturbances and other environmental fluctuations can affect the absolute and relative fitnesses of different species, thereby altering their ability to coexist with one another over the long run.
Previously, I’ve argued at length that ecologists’ best-known ideas about how fluctuating conditions affect competitive coexistence–the cluster of claims called the Intermediate Disturbance Hypothesis (IDH)–are logically flawed zombie ideas, which should be dead but aren’t (see here, here, here, and here). But I’ve only cited, and not explained, the correct way to think about how disturbances and environmental fluctuations affect coexistence (e.g., this post). These correct ideas have mostly been developed in math-heavy papers, making them rough sledding for some people. So I’m going to do a series of posts in which I try to explain, in a way that is both easy to understand yet also accurate, how disturbances and environmental fluctuations actually can promote coexistence.*
I emphasize that I’m not saying anything original in any of these posts. All I’m doing is trying to explain in an accessible way points that have already been made in the primary literature. This post, and the ones to follow, are purely instructional. Indeed, they’re based on how I’ve taught this topic to my undergraduate students for many years. I’m writing for someone who wants to learn the basics of modern coexistence theory but finds the primary literature daunting, not someone who wants to first be convinced that modern coexistence theory is worth learning, or who still needs convincing that zombie ideas about the IDH really are zombies. If you want to argue or push back against the whole edifice of modern coexistence theory, or defend zombies, please hold your fire.
There’s a limit to how much I can explain even in an extended series of posts. I’m going to take for granted that you know some basic mathematics, where by “basic” I mean “simpler than you would typically see in the primary literature in ecology”. I’m going to just focus on mechanisms of coexistence that require environmental fluctuations in order to operate. I’m not also going to explain coexistence mechanisms based on spatial variation, or coexistence mechanisms that can operate independently of spatial variation and temporal fluctuations. And there are of course many technical details I’m going to gloss over, for the sake of explaining key ideas in an accessible way. If you find yourself worried about the missing technical details, you’re of course welcome to inquire in the comments (although if your question is extremely technical or you ask me for lots of details, I’ll probably just refer you to the primary literature, since that’s what the primary literature is there for). I’ll try to remember to conclude the last post in the series with an annotated list of references for those who are interested.
To begin, it’s important to be clear on what is meant by “stable” or “long-term” coexistence. “Stable” coexistence means that each species tends, on average, to increase when sufficiently rare and decline when sufficiently common. It turns out that, if that’s true, and only if that’s true, species will replace themselves on average and so will neither decline to extinction nor increase to infinity.** I think this definition has a lot of intuitive, real-world appeal; it isn’t just some arbitrary abstract assumption theoreticians make for mathematical convenience. After all, in a fluctuating or disturbed environment, any given species is sometimes going to increase, sometimes decrease. It makes sense to distinguish between those temporary blips and their net, cumulative effect on population trends over a longer period of time. So we assume that, if species are truly coexisting, that means that individuals of any given species are replacing themselves on average, which in turn means that species tend on average to bounce back from low density, since otherwise they’d “drift” to extinction. (Note to any readers still spoiling for an argument: if you object to this definition of coexistence on the grounds that what happens on shorter timescales is “ecologically relevant”, or on the grounds that “the long term is difficult to study”, please read this, this, and this and comment over there.)
So, since we’re interested in long-term average behavior, the first thing we need to be able to do is describe and quantify it. Here are the most important mathematical rules for calculating the average value of any quantity that fluctuates over time (actually, they’re the rules for calculating the average of anything, I’m just focusing on their application to time averages). I emphasize that these are mathematical rules, like “2+2=4” or “if a=b, then b=a”; they don’t involve any biology or biological assumptions.
- The average of a constant that doesn’t change over time is just the value of that constant
- The average of x+y equals the average of x plus the average of y
- The average of x-y equals the average of x minus the average of y
- The average of a times x, where a is a constant, is just a times the average of x
- The average of x times y, where x and y both vary over time, equals the average of x times the average of y, plus the population (not sample) covariance of x and y (we’re doing math here, not statistical estimation).
- The average of f(x), where x varies over time and f is some nonlinear function (meaning that if you plot f(x) vs. x you don’t get a straight line), generally does not equal f evaluated at the average value of x. That is, you generally can’t find the average of f(x) by just plugging the average value of x into the function f. For instance, if f(x)=x² and x varies over time, then the average of f(x) equals the square of the average of x, plus the population (not sample) variance of x. Jensen’s Inequality is a well-known special case of this general principle.
It turns out that it’s only a short step from these basic rules of mathematics to some fundamental insights into how disturbance and environmental change affect coexistence.
Let’s say you’ve got some competition model in which species’ per-capita growth rates fluctuate over time, for any reason (disturbance, environmental fluctuations, intrinsically-generated cycles or chaos, demographic stochasticity, whatever). Here’s our first fundamental insight: If you only need to use rules 1-4 to calculate average per-capita growth rates, then fluctuations in your model can’t produce coexistence that wouldn’t otherwise occur.
If all you need to calculate long-term average behavior is rules 1-4, then you have what’s known as a “linear additive” model (this isn’t actually the definition of a linear additive model, but for our pedagogical purposes I think it works fine). That is, you can calculate the average behavior of a linear additive model by just adding (or subtracting) the average values of temporally-varying quantities (rules 1-4), without worrying about “nonadditivity” (rule 5) or “nonlinearity” (rule 6). Which leads immediately to that first key insight, which can be rephrased and elaborated as follows: on average, a linear additive model with temporal fluctuations behaves exactly like an equilibrium model (i.e. a model with no fluctuations), with time-averaged quantities playing the same role as the corresponding constant quantities in the corresponding equilibrium model. Now, this doesn’t mean that a linear additive model with temporal fluctuations can’t have stable coexistence. It’s just that it can’t have stable coexistence that wouldn’t occur in the corresponding non-fluctuating, equilibrium model.
Intuitively, the reason why this occurs is that fluctuations around the average just cancel out in a linear, additive world. In my old “zombie ideas dialogue“, when “Clever Student” talks about a situation in which he and his friend alternately give each other money, he’s talking about a situation in which the flow of money into or out of his pocket fluctuates in a linear, additive fashion.
Here’s a simple, concrete ecological example (I emphasize that it’s merely one deliberately-simple example of the highly-general, indeed universal, principle I just described; don’t mistake the specific example for the general principle). Let’s say you have two species competing for a single shared limiting resource R. Let’s say the per-capita growth rate of one of your competing species equals
(1/N)dN/dt=aeR-m (eqn 1)
where N is its abundance or density, a is the slope of the linear functional response, e is the efficiency of converting consumed resources into new consumers, R is resource abundance or density, and m is the per-capita mortality rate. I’m not going to specify resource dynamics because they don’t matter for the point I want to make.
Important note: a, e, and m generally will differ between species! I just couldn’t indicate that in eqn 1 because I don’t know how to insert proper equations into WordPress and so can’t do subscripts. So in eqn 1, imagine that N, a, e, and m all have subscript i, indicating competitor species i. 😉
In a constant, equilibrium environment, our focal species has a zero per-capita growth rate (i.e. replaces itself, so that eqn 1 equals 0) when R=m/ae (to see this, set eqn 1 equal to 0 and use algebra to solve for R). R=m/ae is the resource level necessary to produce fast-enough births to balance deaths for our focal species. Unless two or more species have precisely identical values of m/ae (which is what mathematicians call “infinitely unlikely”), the species with the lowest value will competitively exclude all the others in the long run; that’s Dave Tilman’s famous “R* rule”. This species reduces the resource to a sufficiently low density, denoted R*, that other species can’t replace themselves. It’s also the only species that can increase when rare in the face of competition from any and all other species. (And even if two species do have identical R* values, then you’ve just got a neutrally stable system which in the real world would result in slow exclusion of all but one species via random drift)
Can environmental fluctuations or disturbances change that and generate stable coexistence? Yes–but it depends how you incorporate them into the model. If you incorporate them in such a way as to produce a linear additive model, then they won’t generate coexistence. For instance, let’s say that the m values fluctuate over time, in any way and for any reason (e.g., because of disturbances or environmental changes) Because the m values fluctuate, species’ per-capita growth rates, and thus their abundances, will fluctuate, causing resource density R to fluctuate as well. But a and e values won’t change, because we’re imagining that whatever causes m values to fluctuate doesn’t change anything else about the competing species (don’t worry about whether that’s empirically realistic or not; remember that I’m explaining conceptual points here). Applying rules 1-4 (we don’t need rules 5-6), the average per-capita growth rate of a focal species will equal
average of (1/N)dN/dt = ae(average R) – (average m) (eqn 2) Notice that the right hand side of eqn 2 looks just like the right hand side of eqn 1, just with the average values of R and m substituted for their previous constant or equilibrium values. In eqn 2, for a species to replace itself on average, the average R must equal that species’ value of (average m)/ae. Whichever species has the lowest value of (average m)/ae will exclude the others in the long run***. In the long run, whichever species is best on average will win–which is exactly the same thing that’s true in a constant environment. Fluctuations in m values around their average values are completely irrelevant to the long-term outcome of competition. This is one specific illustration (far from the only one!) of why Hutchinson (1961) and Huston (1979) were wrong about the IDH. In eqn 2, contra Hutchinson, the frequency with which mortality rates fluctuate is irrelevant. Also contra Hutchinson, it’s irrelevant if mortality rates fluctuate in such a way that different species are competitively superior at different times. And contra Huston, fluctuations in mortality rate due to disturbance events do not slow exclusion by “interrupting” it. What determines the rate of exclusion in a variable or disturbed environment is simply species’ values of (average m)/ae. If they’re similar (which means that species have similar competitive abilities on average), exclusion will be slow. If they’re very different, exclusion will be fast. That’s true no matter how frequently or infrequently sudden increases or changes in mortality rates occur. In a constant environment, species’ values of m/ae determine the rate of competitive exclusion in exactly the same way. Disturbances or environmental fluctuations produce coexistence only via nonadditivity and nonlinearity–basically, via rules 5 and 6 for calculating averages. In future posts, I’ll illustrate this by incorporating environmental fluctuations and disturbance into eqn 1 in different ways. In conclusion, I hope that by explaining things in terms of the mathematical rules for calculating averages, I’m emphasizing the generality of the results I’m explaining. Indeed, I wish I could emphasize it even more, but I don’t know how to do so without getting significantly more technical. I’ve found that many ecologists struggle to grasp just how general and exceptionless modern theoretical work on coexistence is. Many people’s first reaction, if you explain what’s wrong with a specific zombie model like, say, that of Huston (1979), is to question whether there isn’t some other model that nevertheless behaves the way the zombie model is claimed to behave. “Ok, that model doesn’t work–but surely the same mechanism could operate in some other model nobody’s thought of!” Actually, no. In my experience, it’s sometimes hard for folks to understand that’s it’s possible to prove that there is no such model. That certain possibilities–here, certain zombie ideas about how disturbance and environmental fluctuations affect coexistence–really can be ruled out entirely. That, put another way, there are no counterexamples or exceptions to what I’ve said here, or to what I’ll be saying in future posts. The reason we know this is not because theoreticians have analyzed all possible models one by one; that would be impossible. We know it because general mathematical facts, like the rules for calculating averages, let you prove things about the behavior of extremely broad classes of models. Such as “any model in which species exhibit stable coexistence as defined here.” *Then for my next trick I will pull a rabbit out of a hat! 😉 Seriously, it’s really difficult to explain this stuff in a way that’s both accessible and accurate, since dropping math for the sake of accessibility necessarily means reducing rigor. I’m giving it my best shot here, but in all honesty I have no idea how well it will work. When I’m lecturing to undergrads I can pause to chat with them and do question-and-answer, I can use tone of voice and gestures, I can draw lots of diagrams. Blogging is a much more pedagogically-limited medium… **If this strikes you as an empirically-intractable definition, well, it’s not (there are empirical studies estimating species’ average rates of increase when rare). Plus, empirical tractability isn’t my point here. This series of posts is about conceptual clarification. How to make those concepts operational is an important but totally separate issue (to which I may return in a future post). Zombie ideas about how disturbance affects coexistence are notable both for apparently being highly empirically-tractable, and for actually being logically invalid and therefore empirically useless.
Thanks for the post. I have no comments of substance. But regarding mathematical notation in WordPress, I just discovered and used LaTeX in WP for my own blog. It seems to work quite easily:
http://en.support.wordpress.com/latex/
+1 for R code as I find it useful to play with code and visualise ideas whilst taking in their meaning. In your post you can insert LaTeX equations on WordPress.com sites by adding

EQNis the equation you want to typeset in LaTeX notation. Essentially this is standard LaTeX markup but the opening marker is$latexnot simple$. If you aren’t a LaTeX aficionado then switch to Text mode (tab top left of edit window) and insert raw HTML markup(or sup for superscripts).
Hmm, WordPress is too good and has converted my instructions into the LaTeX etc. So, in Text mode, add subscripts by adding this markup:
<sub>i</sub>. For The LaTeX enter
Oh fiddlesticks; just read the link for details on the LaTeX functionality 🙂
Ooo! I didn’t know was handled in comments too. That is nice to know!
was handled in comments too. That is nice to know!
Thanks. I’m unfamiliar with Latex and so would need to try to figure out raw HTML. May give it a go in the next post in this series.
It is actually quite easy to get the hang of in WP (I am a newbie – one post is all I have used it for, and I hadn’t used
in WP (I am a newbie – one post is all I have used it for, and I hadn’t used  previously). For example, the differential equation in your post could be using the code:
previously). For example, the differential equation in your post could be using the code:
$ltx \frac{1}{N}\frac{dN}{dt}=aeR-}$
(but substitute “latex” where I have written “ltx”. I wrote ltx so the code displays; there is sure to be a better way to write the code here – I am a newbie):
code here – I am a newbie):
Use “dfrac” instead of “frac” for larger symbols in the fraction:
Subscripts are simply of the form N_i, so:
$ltx \dfrac{1}{N_i}\dfrac{dN_i}{dt}=a_ie_iR_i-m_i$
would give:
Exponents are of the form x^b:
$ltx x^b$ gives:
Whoops – the bit of code in the first $ltx line should have been:
$ltx \frac{1}{N}\frac{dN}{dt}=aeR-m$
I popped in a } instead of the m.
It’s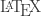 , not Latex… but as many search engines don’t care about case you can get funny results, same thing goes for Twitter (e.g.: https://twitter.com/#!/search/%23LaTeX). So don’t look for
, not Latex… but as many search engines don’t care about case you can get funny results, same thing goes for Twitter (e.g.: https://twitter.com/#!/search/%23LaTeX). So don’t look for 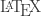 in the presence of children/spouse 😛
in the presence of children/spouse 😛
On Oikos Blog, one of the most common search terms by which people found the blog was “hermaphrodite” or some variation thereon. This was because Chris Lortie once did a post highlighting an Oikos paper on hermaphroditic plants. I’ve always wondered what fraction of people searching on “hermaphrodite” were actually looking for…something other than what they found on Oikos Blog. 🙂
Really nice post, your explanation of Tilman’s R* is great too. I would like to see some R codes that we can play with. Waiting to see how environmental fluctuations can compensate weak stabilizing effects and promote coexistence in part 2. I guess that disturbances would act as an equalizing coexistence mechanism because they do not represent an ecological difference among species.
If you are unfamiliar with generating expressions in Latex you can try the free “daum equation editor” for google chrome or Mac. Make your equation using the tools provided in the app and then copy the Latex form, automatically generated in the bottom of the app.
Glad you liked the post.
Disturbances and environmental change actually can be stabilizing, not (or not just) equalizing. That’s what the future posts will be about.
Hello! Nice post! Did you get to write the others (about disturbances being equalizing vs. stabilizing mechanisms)? I know it has been a while.. Thanks a lot!
Yes, the other posts in the series are linked to in the trackbacks in the comments.
Loved this – glad to get the chance to learn more about linearly-additive models, which I’ve seen you reference before. Hope I get the chance to do some serious math in grad school.
You’ll get a chance to do math if you want to do it. I’m self taught. Haven’t had a math class since high school, except for one probability course in college that I’ve entirely forgotten and never make use of, and a couple of biostats classes which aren’t at all relevant to the stuff I’ve been posting on.
Well, I finished my MS without taking a math class. Rereading this now from today’s Chesson Lesson post, I am really pleased that I understand so much more of it! Grad school wasn’t a waste! 😉 Really, you deserve so much credit for making this material fun yet important.
Thanks Kyle! Glad you found the post helpful. (and congrats on your MSc!)
Pingback: Fighting zombies the old school way | Dynamic Ecology
Pingback: How disturbance and environmental fluctuations actually affect coexistence: part 2 | Dynamic Ecology
Pingback: How disturbance and environmental fluctuations actually affect coexistence: part 3 | Dynamic Ecology
Pingback: Want to bet? | Dynamic Ecology
Pingback: Advice: why should an academic read blogs? | Dynamic Ecology
Pingback: Book review: Community Ecology by Gary Mittelbach, and Community Ecology by Peter Morin | Dynamic Ecology